Maryam spoke about this paper at this week’s combinatorics seminar.

The problem is as follows.  Let be the number of -colourings of a subset of with no monochromatic sum .  What is the maximum of over all ?

One possibility is that we take to be sum-free, so that .  The maximum size of a sum-free set of is around , achieved by the set of odd numbers and the interval , so .

Another possibility is to choose sum-free sets and take all colourings of such that the elements of colour are contained in .  There are

such colourings, where is the number of elements in exactly of the .  For example, we might take half of the to be and half to be .  Then the odd numbers greater than are in every set, and the evens greater than and the odds less than are in half of the sets, so the number of colourings is around

For this matches the previous lower bound; for it is larger.

It’s easy to see that this construction cannot improve the bound for : it only provides good colourings, but as elements contributing to are in , which must be sum-free.

What about ?  Now we get good colourings.  We also have that

But since we have

Moreover, if is not tiny then we are some distance off this upper bound, so the only good constructions in this family come from having all the substantially agree.

How can we get matching upper bounds?  If there weren’t very many maximal sum-free sets then we could say that every good colouring arises from a construction like this, and there aren’t too many such constructions to consider.  This is too optimistic, but the argument can be patched up using containers.

The container method is a relatively recent addition to the combinatorial toolset.  For this problem the key fact is that there is a set of subsets of such that

• every sum-free set is contained in some ,
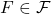• each 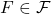is close to sum-free.  Combined with a “sum-removal lemma” this means in particular that it has size not much larger than .

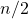We now consider running the above construction with each an element of .  Since the containers are not themselves sum-free, this will produce some bad colourings.  But because every sum-free set is contained in some element of , every good colouring of a subset of will arise in this way.  And since there are most choices for the sets the number of colourings we produce is at most a factor greater than the biggest single example arising from the construction.

This is the big idea of the paper: it reduces counting colourings to the problem of optimising this one construction.  For the authors are able to solve this new problem, and so the original.

In the case where each piece has length and the number of pieces isn’t divisible by , we can’t possibly do better than a maximum difference of between blocks.  Is this achievable in general?

In today’s combinatorics seminar Imre Bárány described joint work with Victor Grinberg which says that the answer is yes.

The proof is algorithmic.  Start with any partition of into parts.  If the difference in length between the smallest and largest parts is at most then we’re done, so assume not.  Fix a longest block and let be a shortest block.  Suppose without loss of generality that is to the right of .  Increase the length of by stealing one piece from the part to the left of .  Repeat this process until either we find a good partition of , or we steal a piece from .  On each iteration a piece moves away from , so one of these must occur in finite time.

At each stage we add a piece of length at most to a block of length more than shorter than , so we never create new blocks of length at least that of .  So when is destroyed there are either fewer blocks of maximal length, or the number of blocks of maximal length has decreased.  There are only finitely many possible lengths for blocks, so by repeating with a new block of maximal length we are guaranteed to find a good partition in finite time.